Alternating Least Squares is a method to Factoring a matrix into two components:
\begin{equation} \mathcal{M}( R) \approx \mathcal{M}(U) \cdot \mathcal{M}(P) \end{equation}
where, we want to come up matricies \(U\) and \(P\) with a certain side length \(k\) that we exdogenously come up with
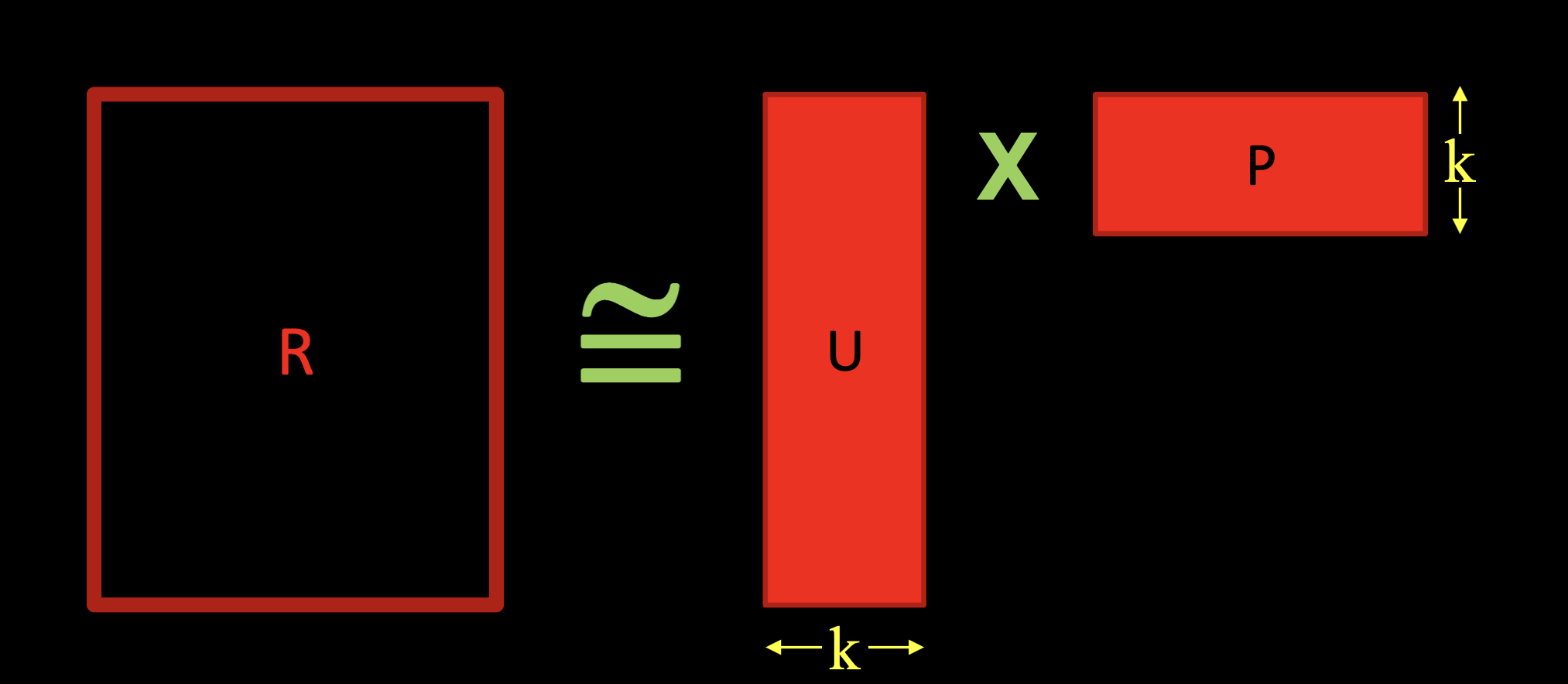
To perform Alternating Least Squares, we fix the values of either \(U\) or \(P\), then perform the least-squares optimization on
(This is proven best-fit for “non-pathological matricies”)